
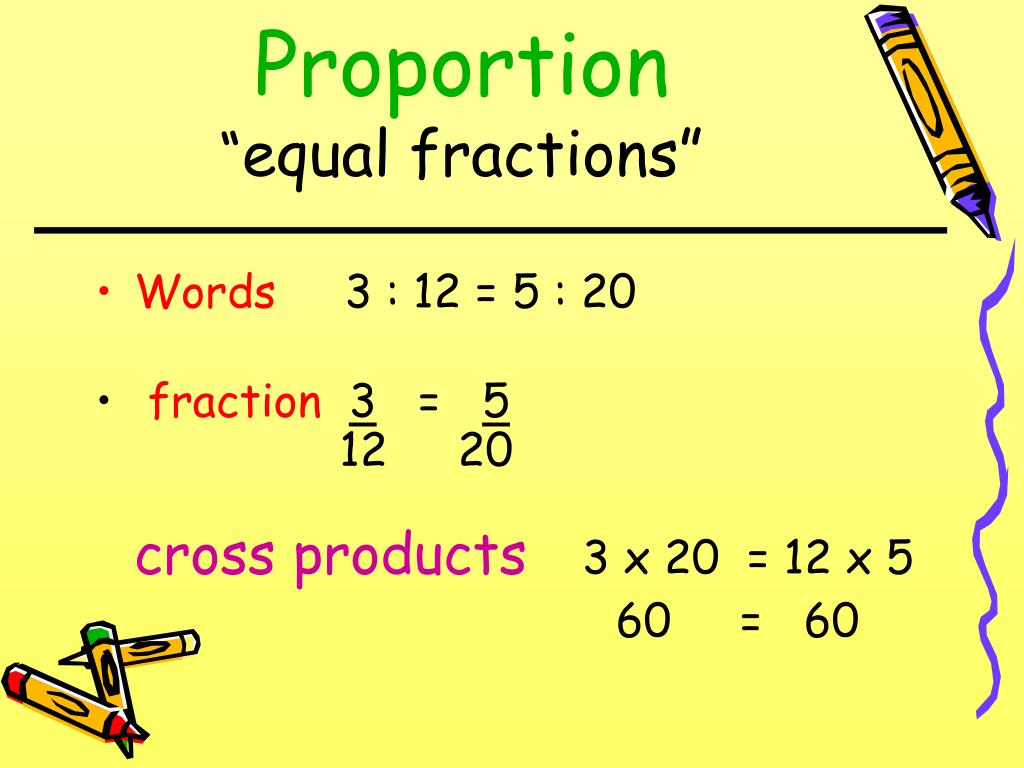
Politicians too can give misleading statements. In newspapers, it is not always clear what quoted percentages are percentages of. A percentage is a percentage of a given quantity: 50% of the voters, 25% of the budget, 10% of the population. = 1.75, which as a percentage is 1.75 × 100% = 175%.Ĭare needs to be taken when talking in percentages. So, for example, 0.17, 0.3 and can be expressed as percentages as follows:ĭecimals or fractions bigger than 1 correspond to percentages greater than 100%. This is a good approach to most indirect proportion problems.įractions and decimals can also be converted to percentages, by multiplying by 100%. It is useful to find out how long it would take one person to do the whole job, then divide by the number of people sharing the work. Such problems can be compared with certain problems involving speed: doubling the number of people working is the same as doubling the speed at which the team work. (An obvious exception to this is decision-making in a committee: if two people can reach a decision in an hour, four people are liable to take twice as long!) The main idea behind this type of problem is that increasing the number of people working decreases the time taken to complete the task. The time taken to do a job is indirectly proportional to the number of people in the team.Ī difficulty with the real-life context of such problems is that, in many cases, it is hard to believe that people working in a team will work at the same rate regardless of the size of the team, unless the team work independently, i.e.
Sometimes as one quantity increases the other decreases instead of increasing. In Section 2.2 you saw that direct proportion described relationships between two quantities, where as one increased, so did the other. So Hicham ran the 1500 metre race faster, which is not surprising as it is a shorter distance. This is a lower speed than 26.21 km per hour. Hence 16.14 miles per hour is the same as 25.99 km per hour. (c) To compare speeds in different units, you need to convert one to the other, say miles per hour to kilometres per hour. To convert to kilometres: divide by 1000 which gives 26.21 km per hour (to 2 d.p.). In 3600 seconds (1 hour) he runs 3600 × metres. 206 seconds), so he ran metres in 1 second. (b) Hicham ran 1500 metres in 3 minutes 26 seconds (i.e. So the athlete's speed is 16.14 miles per hour (to 2 d.p.). 1 hour), he would run 3600/223 miles i.e. Therefore in 1 second he would run 1/223 miles. In 223 seconds (3 minutes 43 seconds) he ran 1 mile. (a) To find the speed in miles per hour, you need the ratio of the distance in miles to time in hours.


 0 kommentar(er)
0 kommentar(er)
